Imagine it is game four of the ALCS, and the Boston Red Sox are up three games to none over the New York Yankees. A smart bettor might choose to place his game four bet on the Yankees, citing the fact that they would never allow the Red Sox a clean sweep and are due for a win. One might compare betting on the Yankees in this situation to betting on “heads” after three consecutive “tails” coin tosses. However, the baseball bettor is in an infinitely better position than the coin-tosser. Unlike a coin toss, a baseball game is not a chance event. There are many factors contributing to baseball (e.g., team morale, player strengths and weaknesses, home field advantage, etc.) that can lead to a more informed1 bet. The coin-tosser is left only with the odds (i.e., 50/50) and player’s intuition—the latter of which often becoming a liability rather than an asset. Several recent WAGERs have examined the events and processes that shape gamblers’ decision-making (e.g., WAGERs 8(43) and 9(14)); this week’s WAGER expands on this topic with a look at the relationship between the gambler’s fallacy and event sequence. Specifically, Roney and Trick (2003) examined whether peoples’ natural tendencies to identify patterns and group events (e.g., a series of “heads” tosses) into broader units alters gamblers’ perceptions of the odds and resulting gambling behaviors.
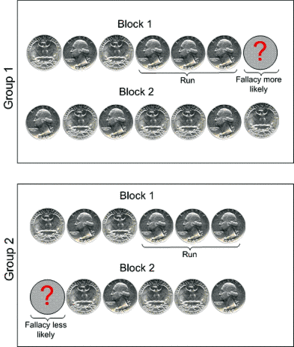
Roney and Trick (2003) describe the gambler’s fallacy as a subject’s “tendency to erroneously believe that for independent events, recent or repeated instances of an outcome (e.g., a series of “heads” when flipping a coin) will make that outcome less likely on an upcoming trial” (p. 69). To test the whether this phenomenon is related to grouping (i.e., whether an event’s inclusion in an event series influences a gambler’s tendency to commit the fallacy), the authors enrolled 127 psychology students at a Canadian University to participate in a study of coin toss grouping. Investigators separated participants into experimental groups (i.e., no more than thirty subjects per group) and assigned experimental groups to one of two conditions: six coin tosses per trial block or seven coin tosses per trial block (i.e., event series; see Figure 1). Participants were unable to see the outcome of each coin toss from their vantage point. The first three tosses in each block were random and reported to participants truthfully; however, the fourth, fifth and sixth tosses were always reported to participants as a run (i.e., three consecutive heads or tails). Thus, each subject’s answer to the seventh (i.e., “critical”) toss determined whether they committed the fallacy. Depending on group assignment, this critical toss occurred either as the last trial in block one (i.e., groups with seven tosses per block), or the first trial in block two (i.e., groups with six tosses per block; see Figure 1). The authors asked participants in each group to predict the outcome of the seventh toss and make bets (up to $1.00) on their prediction. Subjects also indicated their confidence in each of their bets on a 7-point Likert scale.
Figure 1. Coin toss structure among groups (Roney & Trick, 2003)
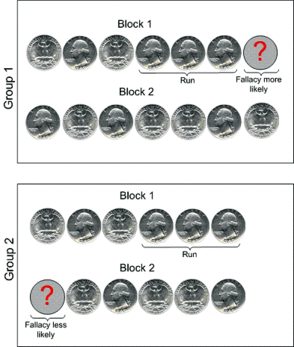
Roney and Trick (2003) found that subjects in the seven-toss block groups (i.e., Group 1) were significantly more likely to predict a discontinued run (i.e., commit the fallacy; X2 (1, 126) = 17.91, p<.01)) than subjects in the six-toss block groups (i.e., Group 2). Subjects in Group 1 also placed higher bets and exhibited more confidence on the critical toss than subjects in Group 2 (amount bet, t(123) = 5.15, p<.001; confidence, t(124) = 4.82, p<.001). These results supported their hypothesis that the gambler’s fallacy is affected by subjects’ natural tendency to organize events into larger, cohesive units. When the critical toss was part of the natural unit (i.e., Block 1) the fallacy prevailed; however, when the critical toss occurred in a new, separate unit (i.e., Block 2), the fallacy became less common.
While interesting on a theoretical level, several methodological limitations limit the applicability of these results to real gambling situations. For example, subjects did not place bets with real money; it is unclear whether they would have behaved similarly had they been playing with their own funds. In addition, the investigators did not screen subjects for gambling problems. As a result, it is impossible to know whether disordered gamblers would exhibit the same gambling behaviors as the subjects in this study. Further, subjects in this study tracked the coin toss results on paper, whereas casino gamblers generally do not. Lack of precise information about their win/loss patterns might cause casino gamblers to act differently than the current sample. Finally, subjects were limited in the total number of bets they were allowed (i.e., 12 or 14). Because the study design did not educate participants about the hazards of the fallacy (but simply encouraged Group 2 subjects to detach themselves from the previous sequence), it is unclear whether subjects in Groups 1 and 2 would have exhibited different behaviors (and fared any differently) over a long betting session.
Despite these concerns, the results of this study have several implications for clinicians and educators. First, and perhaps most importantly, this study indicates a greater need for public education regarding the statistics of gambling. Greater familiarity with the gambler’s fallacy and its pitfalls will ultimately help gamblers of all types make healthier decisions. These results also suggest that encouraging gamblers to take breaks more frequently might discourage fallacy-induced betting. Because gamblers were less likely to fall into the fallacy when the critical toss began a new series (i.e., Group 2 scenario), this suggests that frequent breaks might allow gamblers an opportunity to detach themselves from previous betting sessions by breaking the subjective “Gestalt” and approach additional betting more objectively.
Comments on this article can be addressed to Tony Donato.
Notes
1 This is not to suggest that additional information will guarantee a successful bet. Although certain betting situations (e.g., horseracing, sporting events) afford the bettor the opportunity to incorporate outside information when considering a wager, there is no such thing as a “sure” bet or guaranteed win.
References
Roney, C. J., & Trick, L. M. (2003). Grouping and gambling: A Gestalt approach to understanding the gambler’s fallacy. Canadian Journal of Experimental Psychology, 57(2), 69-75.